In a DC system, the voltage and current are constant, therefore, there is no problem in specifying their magnitude. But in the case of an AC system, an alternating voltage varies from instant to instant and so poses a problem in specifying the magnitude.
 
So to express the AC quantities in an easy and understandable form, the concept of RMS value has been developed. They also can be expressed in terms of peak value or average value but these values don’t express the effectiveness of AC quantity.

Effective or RMS value of AC Current

The RMS value of AC current is equal to that amount of DC current which produces the same heating effect flowing through the same resistance for the same time.
 
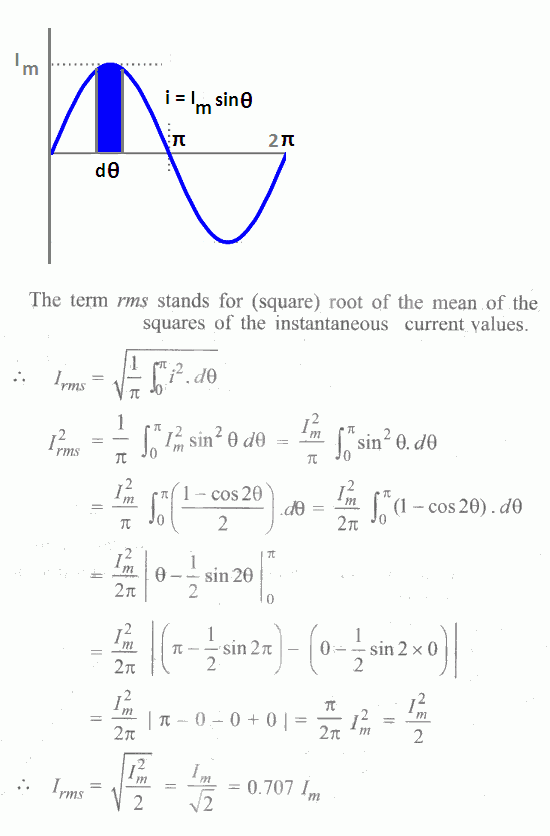
The term RMS stands for the (square) root of the mean of the squares of instantaneous current values. The RMS value of AC current or voltage can be calculated from the following relation: 
 
I_RMS  =  0.707 x peak value of current
V_RMS =  0.707 x peak value of voltage
 

It is the actual value of an alternating quantity that tells us the energy transfer capability of an AC source. For example, if we say that 5A alternating current is flowing through a circuit, it means the RMS value of AC current flowing through the circuit is 5A. And it will produce the same amount of heat (energy) as will be produced by 5A DC current.
 
All the AC ammeters and voltmeters show the RMS values of alternating current and voltage respectively. All the electrical appliances are rated in the terms of RMS value. In India, the domestic single-phase AC supply is 230 V, 50 Hz. Here, 230 V is the RMS value of the supply voltage.
 
The RMS value of AC is always greater than the average value except for a rectangular wave when these are equal. Its value can never be negative.

Drive the Expression for RMS Value of AC Current

Let i = I_m sinωt be the alternating current flowing through a resistance of R ohms for time t seconds and produces the same heat as produced by I_eff (a direct current).
 
The base of the half-cycle is divided into n equal parts as shown in Figure, so that each interval is of t/n seconds.

 
Let i₁, i₂, i₃ ……….i_n be the mid-ordinates.
 
Then, heat produced in first interval    = i₁²Rt/n  joules
                               Second  interval    = i₂²Rt/n   joules
                               Third interval        = i₃²Rt/n  joules
                               n^th  interval          = i_n²Rt/n  joules
 
Total heat produced in time t
 
=  Rt[(i₁² + i₂² + i₃² + ……+ i_n²)/n]  …..(1)
 
Since I_eff is considered as the effective value of this current.
Then heat produced by this current in time t = I_eff²Rt …….(2)
 
By definition, equations (1) and (2) are equal. Therefore,
 
I_eff²Rt = Rt[(i₁² + i₂² + i₃² + ……+ i_n²)/n] 
 
Or  I_eff² = [(i₁² + i₂² + i₃² + ……+ i_n²)/n] 
 
Or  I_eff = [(i₁² + i₂² + i₃² + ……+ i_n²)/n]^1/2 
 
Or I_eff = I_RMS = square root of the mean of the squares of the instantaneous current.
 
Now, by using the integral calculus the RMS or effective value of the alternating current over a time period can be calculated as follows: 

Important Terms Related to RMS Value of AC

Peak Value (I_m): The maximum value attained by an alternating current during a complete cycle is called its peak value. A sinusoidal alternating current attains its maximum positive value at 90^o.
 
Form Factor (K_f): It is the ratio of the RMS value to the average value of alternating quantity.
 
K_f = RMS value/ Average value
 
For a sinusoidal wave, it is 1.11. We can find the RMS value from the average value and vice-versa with the help of the Form factor.
 
This factor shows the “peakiness” of the waveform. A square wave that is flatter than a sine wave, has a form factor of 1.0. On the other hand, a triangular wave is more “peaky” than a sine wave and has a form factor of 1.15.
 
Peak Factor (Kp): It the ratio of the peak or maximum value to the RMS value of an alternating quantity.
 
K_p = Peak value / RMS value
 
For a sinusoidal wave, it is 1.414. It is very significant in dielectric insulation testing because the dielectric stress on the insulation material depends upon the peak value of the applied voltage. It is also important while measuring the iron losses because these losses depend on the value of maximum flux.

Average Value of AC Current

The arithmetic average of all the instantaneous values considered in an alternating quantity current over one cycle is called the average value of AC Current (I_av).
 
It is that steady current (DC) that transfers the same charge as transferred by the AC current in same time across any circuit.
 
In the case of symmetrical waves like sinusoidal current or voltage wave, the positive half is exactly equal to the negative half, therefore, the average value over a complete cycle is zero.
 
Since work is being done by the current in the positive as well as in the negative half cycle, therefore, the average value is determined regardless of signs, or only the positive half is considered.
 
To determine, the average value of alternating current, consider only in the positive half cycle and divide it into n number of equal parts. Let i₁, i₂, i₃ ……in be the mid-ordinates.
 
Now, average value of current, I_av = Mean of mid-ordinates
 
= (i₁ + i₂ + i₃ + …….+i_n)/n
 
= Area of half cycle/length of base of half cycle
 
Now, by using the integral calculus, we can calculate the area of half-cycle and the average value of the alternating current.  After this calculation, we get the following expression for the sinusoidal current.
 
I_av = 2I_m/π = 0.637 I_m
 

• AC Fundamentals
• RMS value of AC Current
• Purely Resistive | Inductive | Capacitive Circuit
• RL | RC | RLC Series Circuits
• Power Factor in AC Circuit
• Power Factor Improvement Using Capacitor Bank
• Star Connection
• Delta Connection
• Power Measurement in Three Phase Circuits

© https://www.yourelectricalguide.com/ rms value of ac derivation.